[10 min, via Thread]
Over the past 20 years, my stupid, grasping mind has trying to find ways to combine music and cosmology. This began with writing music that I (and others) have struggled to describe verbally.
When I first stumbled into the methods of creating this highly textural form, I knew I’d laid the foundations for all the music I would write in the future. It was a moment I found so exciting I had to immediately find a phone box (yes, that long ago) to call my friend Andy to tell him. We have collaborated ever since.
For me, music, like mathematics, is a non-verbal language. It is experiential.
A theme for me seems to be to begin with creating common languages. This work has evolved to explore the articulation and progression in musical language, one that has mirrored our understanding of the universe for centuries. I’ve tried to show this evolution, in broad terms, in the image above.
We used to believe there was a fundamental relationship between the distances between the planets and music. We called this Musica Universalis or ‘Music of the Spheres’. We know now there isn’t a causal relationship, but our emotional relationship with this idea remains profoundly powerful.
My question was ‘is there an equivalent in contemporary physics and electronic music?’. Is there a ‘Music of the Hypersphere’? Or, bringing in more specific language, is there an ‘Acoustic cosmology in Hilbert Space’. You might also ask ‘What is the shape of music?’.
My explorations led me to try and find relationships (mathematical, or experiential, not causal) between the mathematics used in astrophysics (specifically cosmology and quantum mechanics) and the codified mathematics-as-algorithms in the realms of computer- and electronic-music. For those who understand science and/or music, some of this will make no sense in either of those domains. This is intended: we are exploring. This isn’t a small project.
We can draw interesting parallels between the macro-languages of music over centuries and the evolution of our understanding of the complexity of the universe.
Starting about a thousand years ago, with PITCH and DURATION in monophonic music (polyphony was considered ungodly), through to the development of HARMONY in baroque to the ROMANTIC and COMPLEX symphony into REDUCTIONIST music-concrete, and the ATOMISED computer-music to the contemporary GENERATIVE algorave, we can see clear parallels between the language used to describe our perception of the universe and the words used to describe music.
Our latest iterations can reduce the sonic universe (soniverse) to a set of fundamental equations (the equivalents to Maxwell’s equations) from which all other sounds can be produced. From additive synthesis to neural network-generated chart hits, it seems to me that these very different worlds form part of our convergence of understanding: art and science are reconvening.
This had led me to ask: is there a musical equivalent to the CURVATURE of space-time? Can we operate natively in a frequency, rather than a time-based domain? What might DIMENSIONALITY mean in a wave-time cosmology?
My hypothesis is to test if there exists and/or if we can create a soniverse in which the language of science is relevant. It may be relevant in a causal sense (for example, the physics may be self-referentially consistent). It may be relevant aesthetically (for example, it may describe a subjective musical construct). Our first principle is to create a fundamental particle, which I will call a sonon — the equivalent to a photon in this sonic universe (and not to be confused with a phonon). Our next challenge is to start to define its properties, and then to define the physics that may apply to this universe.
Our single sonon is one wavelength. Time is, as yet, undefined.
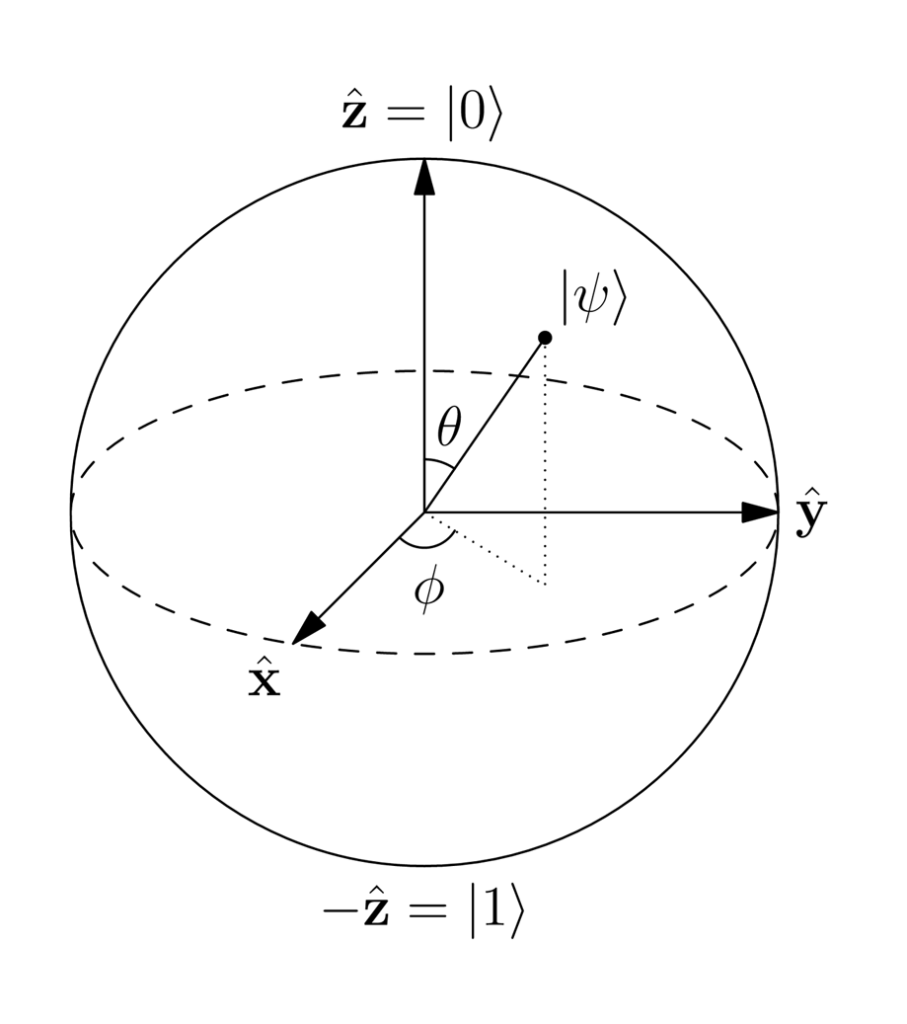
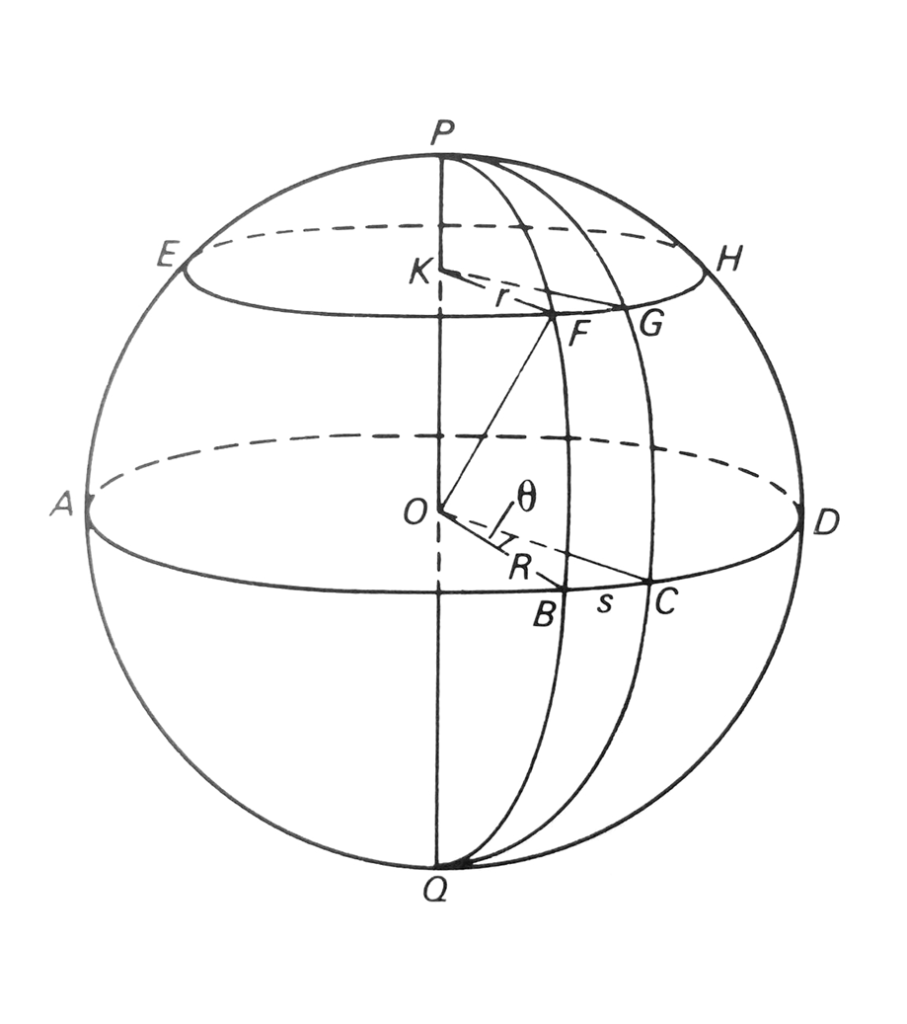
There is a lovely visual similarity between how navigators and astronomers have been mapping the heavens for centuries (the Celestial Sphere), and the way scientists model quantum mechanics (the Bloch Sphere).
Whereas a Celestial Sphere helps us map the entirety of the heavens by imagining you as the observer (O) at the centre of the universe, a Bloch Sphere is a geometric representation of the complex mathematics of qubits, used in quantum computing. In ‘normal’ computing, a bit is either a one or a zero. A qubit can kind of be both at the same time (see “you guys put complex numbers in your ontologies?”). And if that sounds beautifully confusing, it is.
As I’ve been looking at these ways of viewing the universe at such wildly different scales, and how Bloch Spheres are used to help describe photons, I wanted to attempt to create an equivalent of a Bloch Sphere to define dimensions of influence in our sonon. As I’m also trying to bring in the large-scale cosmological mathematics, it seemed to fit to deal with these confusing extremes in parallel.
We begin with phase in a classical sense. We then consider amplitude to be defined by the number of sonons counted (as classical physics, we’ve not yet considered quantum state probability amplitudes). In sound, as classically defined in our universe as a pressure wave, there is no polarisation. This makes it hard to create any mapping that might lead to concepts of entanglement, or that captures the strangely observer-centric nature of photons.
Instead, we create the notion of temporal musicality. A sonon is and is not musical if it is embodied in an observable frame of reference that is musical (e.g. an observable frame of reference could be a piece performed in a rendered space for a listener). We define ‘musical’ as ‘an emotive response’ over a period of time (performance time). A sonon may be considered musical if it lies on the surface of the Bloch Sphere. Inside the Bloch Sphere, we admit that we are not sure if it is.
Whether or not it is, is dependent on its observation—which embodies the entire context and structure in which it is heard, and the listener. It is impossible to recreate a sonon since it is, by definition, only rendered in a temporal space as an auditory event.
We may or may not be able to model context: a combination of sonons in an acoustic environment. We may or may not be able to model the listener: which could range from a microphone to a human, from a bird to a black hole, and therefore may be unknowable. We may be unable to differentiate between a sonon and a rendered sonon (e.g. if time runs backwards, is it a different sonon?).
We create a parallel of wavefunction collapse as this temporal rendering. A sonon can exist in many states prior to being heard. It is only the act of hearing that renders it as having a musical response (cf. in quantum mechanics it is not possible to know the state of a photon until it is observed). (I leave the idea that consciousness is a prerequisite for ‘hearing’ as an exercise for another day. It’s also interesting to think about how you might render Cage’s 4’33” in this context and whether a sonon could be considered musical without any other context.)
Having defined a fundamental particle, we then consider what physics may exist in this soniverse. We first must create time. We are drawn to ideas of ‘causality’: in this context, causality is only rendered by the listener. Our soniverse may end up only being rendered temporal through experiencing it. It is multi-directional: conversations can be run backwards as well as forwards.
Our big-bang event is the spontaneous creation of all sonons that can exist. We expand the event horizon of the soniverse exponentially and introduce spontaneous random variations. Our parameters of expansion are time, phase and wavelength. We do not define any physical space (there is no space-time). In our initial model, distance is only measured in sonon wavelengths. Analogous to the relativistic perspective of a photon, its position is ‘everywhere’ and its ‘distance’ is ‘nothing’ in between. It may be that we begin in zero or one dimension.
We define Time to have three independent dimensions: the individual sonon wavelength (=1?), the duration an individual instrument as rendered (the instrument time), and a duration equal to the length of an individual piece (the performance time). An instrument time can be longer than a performance time (for example, the 1000 year piece Longplayer, could be considered transitory: an individual’s snippet of conversation could be considered a shard of the instrument that is every word they ever utter).
In our wave-time, wavelength, phase and Time may vary over time. We define an equivalent of the inverse square law: sonons diminish to the square of their wavelength. We define a temporal gravity whereby harmonics may cluster at intervals proportional to their relative density at temporal wavelengths (ie. repetition of sonons within a localised event horizon may cause clustering—’mass’). This could, for example, be an equivalent to a ‘rhythmic or musical phrase’ in classical music. Sonons attract if there is a temporal ‘harmony’.
We define an Epoch to be the finite age of this soniverse. It is equal to or longer than the performance time.
We define a curvature of wave-time. This curvature is in frequency space (Sμʊ;ג) and is time-variant based on where we are in this epoch.
We can also attempt to visualise the soniverse in our four-dimensional physical space-time. We can develop new modes of listening, and new modes of interaction between these two universes.
We can take an individual sonon cluster and redshift it. We can take clusters of our virtual sonons and vary their localised gravity based on where our ‘projected hand of god’ creates motion in a sonon cluster.
We can enquire if there is a concept of ‘aubit’ — the equivalent of a physical orbit. For example, if we can define the notion of ‘clustering’, then we can define clustering in relation to a notion of gravitation that operates in a cosmological context (the wave-time is bent in the soniverse as space-time bend in the universe).
We can create singularities: supermassive, hyper-localised gravity that acts to distort the surrounding wave-time akin to gravitational lensing. A listener may traverse a timeline in any direction.
We can define entropy and declare it to increase and decrease as the soniverse expands and contracts. Entropy does not really derive from the expansion/contraction of the universe (they are related, but not consequential) but I have always assumed them to be quite deeply linked. I couldn’t explain to you why, but I like the idea of either decay-to-noise, or crystallisation-from-noise, and to me, these are linked to the idea of big bang and big crunch in an oscillating universe.
In the beginning there was nothing. And a god said, “Let there be noise”.
We have yet to define other particles, such as electrons or quarks, or other atomic structures. If I was an academic, at this point I would say something like ”we leave molecular interactions as an exercise for the reader”, however, I’m much more interested in the actions below.
We’ve started modelling this here: http://www.astro.ljmu.ac.uk/~amn/Soniverse/demograph.html
Actions
- If you have no idea about any of the science, I’d love to hear your feedback about the ideas you took away from reading this. Over time I want to find ways of trying to write about this in an accessible way.